Question

Solve the inequality. Write your answer in Interval Notation. Show your work.

4 ( x + 2 ) - 5x < x + 20

Answer 1

(-6, +∞)

Explanation:

4(x + 2) - 5x < x + 20 Distribute the 4

4x + 8 - 5x < x + 20 combine like terms

-x + 8 < x + 20 Add x to both sides

-x + x + 8 < x + x + 20

8< 2x + 20 Subtract 20 from both sides

-12 < 2x Divide both sides by 2

`(-12)/(2)` <
`(2x)/(2)`

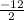-6 < x

x > -6

My solutions are all the numbers larger than -6.

Helping in the name of Jesus.

Answer 2

x > -6

Detailed explanation:

Hi there! Our task is to solve the inequality.

First, use the distributive property:

`\mathbf{4(x+2)-5x < x+20}`

Combine like terms:

`\mathbf{4x+8-5x < x+20}`

`\mathbf{-x+8 < x+20}`

Subtract x from each side:

`\mathbf{-x-x+8 < 20}`

Combine like terms:

`\mathbf{-2x+8 < 20}`

Subtract 8 from each side:

`\mathbf{-2x < 20-8}`

`\mathbf{-2x < 12}`

Reverse the inequality sign:

`\mathbf{x > -6}`

`\bigstar` Therefore, x > -6.