Final Answer:
The maximum area of a triangle formed in the first quadrant by the
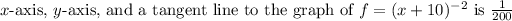 square units.
square units.
Step-by-step explanation:
To find the maximum area of the triangle formed by the given function, we need to consider the tangent line that intersects the graph in the first quadrant. The function
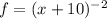 represents a hyperbola, and its tangent line in the first quadrant will be parallel to the y-axis.
represents a hyperbola, and its tangent line in the first quadrant will be parallel to the y-axis.
Let's denote the point of tangency as
 The slope of the tangent line is given by the derivative of the function evaluated at
The slope of the tangent line is given by the derivative of the function evaluated at
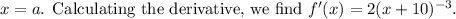 Setting
Setting
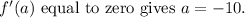
Now, the base of the triangle is twice the x-coordinate of the point of tangency, so
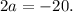 The height of the triangle is the y-coordinate of the point of tangency, which is
The height of the triangle is the y-coordinate of the point of tangency, which is
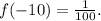 Therefore, the area
Therefore, the area
 is given by
is given by
 .
.
Thus, the maximum area of the triangle is
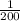 square units.
square units.