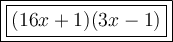Answer:
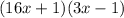
Explanation:
Given quadratic:
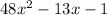
To factor a quadratic in the form ax² + bx + c, we first need to find two numbers that multiply to ac and sum to b.
The coefficients of the given quadratic are:
Therefore, the product of a and c is -48.
The factors pairs of -48 are:
- 1 × -48
- -1 × 48
- 2 × -24
- -2 × 24
- 3 × -16
- -3 × 16
- 4 × -12
- -4 × 12
- 6 × -8
- -6 × 8
Therefore, the two numbers that multiply to -48 and sum to -13 are 3 and -16.
Rewrite b as the sum of these two numbers:
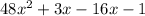
Factor the first two terms and the last two terms separately:
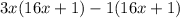
Factor out the common term (16x + 1):
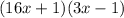
Therefore, the given quadratic factored is: