Question

A projectile is launched upward with a velocity of 160 feet per second from the top of a 30-foot stage. What is the maximum height attained by the projectile?

Answer 1

The maximum height attained by the projectile is 530 feet.

Step-by-step explanation:

The maximum height attained by the projectile can be determined using the equations of motion. The vertical motion of the projectile can be described by the equation h = v02 / (2g) + h0, where h is the maximum height, v0 is the initial vertical velocity, g is the acceleration due to gravity, and h0 is the initial height. Plugging in the given values, we get h = (1602) / (2 * 32) + 30 = 530 feet.

Answer 2

The maximum height attained by the projectile,. given that it was launched from the top of a 30-foot stage is 430 feet

How to calculate the maximum height?

First, we shall obtain the height attained by the projectile from the the top of the stage. Details below:

• Initial velocity of projectile (u) = 160 feet per second
• Final velocity of projectile (v) = 0 feet per second (at maximum height)
• Acceleration due to gravity (h) = 32 feet per second square
• Height attained from the stage (h) = ?

`h = (u^2)/(2g) \\\\h = (160^2)/(2\ *\ 32) \\\\h = 400\ feet`

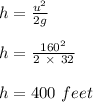
Finally, we shall calculate the maximum height of the projectile. Details below:

• Height of stage = 30 feet
• Height attained from the stage = 400 feet
• Maximum height of projectile =?

Maximum height of projectile = Height of stage + Height attained from the stage

= 30 + 400

= 430 feet