The blood speed in the aorta is approximately 0.296 meters per second (m/s).
The total cross-sectional area:
A_total = A_capillary * N_capillaries = π * (4 * 10^-6 m)² * 7 *
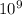
= 70.4π *
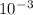 m²
m²
The Flow rate = A_total * v_capillary
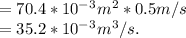
The flow rate through the aorta must be equal to the total flow rate through the capillaries, assuming no blood loss throughout the circulatory system.
The cross-sectional area of the aorta
A_aorta = π * (10.9 *
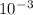 m)²
m)²
= 118.15π *
 m²
m²
Finally the blood speed: v_aorta = Flow rate / A_aorta
= (35.2π *
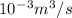 ) / (118.15π *
) / (118.15π *
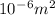 )
)
= 0.296 m/s.