Question

An observer is 560 m horizontally from the launch pad of a rocket. After the rocket has ascended 710 m, how far is it from the observer? The distance between the observer and the ascended rocket is m. (Round to the nearest whole number as needed)

Answer 1

≈ 904 m

Explanation:

We can use a right triangle to model the situation

the horizontal leg is 560, the vertical leg is 710, the height of the rocket and the hypotenuse is the distance from the observer to the ascended rocket.

using Pythagoras' identity in the right triangle.

the square on the hypotenuse is equal to the sum of the squares on the other 2 legs.

let d be the distance from the observer to the ascended rocket , then

d² = 560² + 710² = 313600 + 504100 = 817700

take the square root of both sides

d =
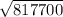`√(817700)` ≈ 904 m ( to the nearest whole number )