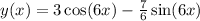Answer:
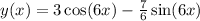
Explanation:
Solve the roots
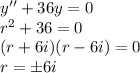
Because the roots are complex conjugates, then the general solution we'll need to use is
![y(x)=e^(\alpha x)[c_1\cos(\beta x)+c_2\sin(\beta x)]](https://img.qammunity.org/2024/formulas/mathematics/high-school/qedgt0328sr5xcpkpqhc18ffwow261grqw.png) where
where
 .
.
This makes the general solution
![y(x)=e^(0x)[c_1\cos(6x)+c_2\sin(6x)]=c_1\cos(6x)+c_2\sin(6x)](https://img.qammunity.org/2024/formulas/mathematics/high-school/m5lq1ojdxg53rzffcxvkpf89gi36wsamw7.png)
Since
 , we can plug in our initial conditions into
, we can plug in our initial conditions into
 and
and
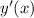 to solve for each constant:
to solve for each constant:
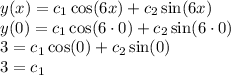
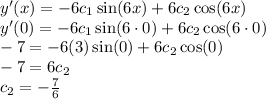
Therefore, the solution to the given IVP is