Question

!50 POINTS! (3 SIMPLE GEOMETRY QUESTIONS)

QUESTIONS BELOW
|
|
\/

Answer 1

1) 135°

2) 133

3) 72

Explanation:

Question 1

To determine the interior angle of a regular polygon, we can use the formula:

`\boxed{\theta= (180^(\circ)(n-2))/(n)}`

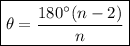
where:

• θ is the interior angle.
• n is the number of sides.

Given the number of sides of a regular octagon is 8, substitute n = 8 into the formula:

`\begin{aligned}\implies \theta&= (180^(\circ)(8-2))/(8)\\\\&= (180^(\circ)(6))/(8)\\\\&= (1080^(\circ))/(8)\\\\&=135^(\circ)}\end{aligned}`

Therefore, the angle is 135°.

`\hrulefill`

Question 2

The given diagram shows an isosceles trapezoid, since its legs are equal in length.

Opposite angles in an isosceles trapezoid are supplementary (sum to 180°). Therefore:

`\begin{aligned} x^(\circ)+47^(\circ)&=180^(\circ)\\x^(\circ)+47^(\circ)-47^(\circ)&=180^(\circ)-47^(\circ)\\x^(\circ)&=133^(\circ)\\x&=133\end{aligned}`

Therefore, the value of x is 133.

`\hrulefill`

Question 3

The given diagram shows an isosceles trapezoid, since its legs are equal in length.

Opposite angles in an isosceles trapezoid are supplementary (sum to 180°). Therefore:

`\begin{aligned} x^(\circ)+108^(\circ)&=180^(\circ)\\x^(\circ)+108^(\circ)-108^(\circ)&=180^(\circ)-108^(\circ)\\x^(\circ)&=72^(\circ)\\x&=72\end{aligned}`

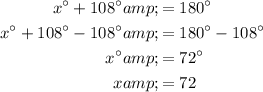
Therefore, the value of x is 72.