Question

The current in the long, straight wire AB shown in the figure is upward and is increasing steadily at a rate di/dt.a. What is the induced emf in the loop?b. Evaluate the numerical value of the induced emf if a = 12.0 cm, b = 36.0 cm, L = 24.0 cm and di/dt = 9.60 A/s.

Answer 1

The induced emf in a loop is given by the product of the rate of change of magnetic flux through the loop and the number of turns in the loop (Faraday's law of electromagnetic induction).

Step-by-step explanation:

The induced emf in a loop is given by the product of the rate of change of magnetic flux through the loop and the number of turns in the loop (Faraday's law of electromagnetic induction). In this case, the loop is perpendicular to the magnetic field created by the current in the wire AB, so the magnetic flux through the loop is changing due to the changing current. The induced emf can be calculated using the formula:

emf = -N(dΦ/dt)

where N is the number of turns in the loop, and (dΦ/dt) is the rate of change of magnetic flux.

Answer 2

`\[ \]\[ B = \frac{(4\pi * 10^(-7) \, \text{T m/A}) \cdot i}{2\pi \cdot r} \]`

`\[ \]\[ \Phi = \left(\frac{(4\pi * 10^(-7) \, \text{T m/A}) \cdot i}{2\pi \cdot r}\right) \cdot (0.12 \, \text{m} \cdot 0.24 \, \text{m}) \]`

`\[ \]\[ \text{Total Flux} = \int_(0)^{0.36 \, \text{m}} \left(\frac{(4\pi * 10^(-7) \, \text{T m/A}) \cdot i}{2\pi \cdot r}\right) \cdot (0.12 \, \text{m} \, dx) \]`

`\[ \]\[ \varepsilon = -(d)/(dt) \left( \int_(0)^{0.36 \, \text{m}} \left(\frac{(4\pi * 10^(-7) \, \text{T m/A}) \cdot i}{2\pi \cdot r}\right) \cdot (0.12 \, \text{m} \, dx) \right) \]`

**(a) Magnetic Field
`(\( B \))`:

`\[ B = (\mu_0 \cdot i)/(2\pi \cdot r) \]`

**(b) Magnetic Flux
`(\( \Phi \))` through the Narrow Strip:**

`\[ \Phi = B \cdot A = B \cdot (a \cdot L) \]`

**(c) Total Flux through the Loop:**

`\[ \text{Total Flux} = \int_(0)^(b) B \cdot (a \, dx) \]\[ \text{Total Flux} = \int_(0)^(b) \left((\mu_0 \cdot i)/(2\pi \cdot r)\right) \cdot (a \, dx) \]`

**(d) Induced EMF
`(\( \varepsilon \))`in the Loop:**

`\[ \varepsilon = -(d)/(dt) \left( \int_(0)^(b) B \cdot (a \, dx) \right) \]`

Now, substitute the given values:

`- \(a = 12.0 \, \text{cm} = 0.12 \, \text{m}\)- \(b = 36.0 \, \text{cm} = 0.36 \, \text{m}\)- \(L = 24.0 \, \text{cm} = 0.24 \, \text{m}\)- \((di)/(dt) = 9.60 \, \text{A/s}\)- \(\mu_0 = 4\pi * 10^(-7) \, \text{T m/A}\)`

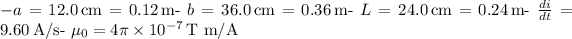
**(a) Magnetic Field
`(\( B \))`:**

`\[ B = (\mu_0 \cdot i)/(2\pi \cdot r) \]\[ B = \frac{(4\pi * 10^(-7) \, \text{T m/A}) \cdot i}{2\pi \cdot r} \]`

**(b) Magnetic Flux
`(\( \Phi \))` through the Narrow Strip:**

`\[ \Phi = B \cdot (a \cdot L) \]\[ \Phi = \left(\frac{(4\pi * 10^(-7) \, \text{T m/A}) \cdot i}{2\pi \cdot r}\right) \cdot (0.12 \, \text{m} \cdot 0.24 \, \text{m}) \]`

**(c) Total Flux through the Loop:**

`\[ \text{Total Flux} = \int_(0)^(b) \left((\mu_0 \cdot i)/(2\pi \cdot r)\right) \cdot (a \, dx) \]\[ \text{Total Flux} = \int_(0)^{0.36 \, \text{m}} \left(\frac{(4\pi * 10^(-7) \, \text{T m/A}) \cdot i}{2\pi \cdot r}\right) \cdot (0.12 \, \text{m} \, dx) \]`

**(d) Induced EMF
`(\( \varepsilon \))` in the Loop:**

`\[ \varepsilon = -(d)/(dt) \left( \int_(0)^(b) B \cdot (a \, dx) \right) \]\[ \varepsilon = -(d)/(dt) \left( \int_(0)^{0.36 \, \text{m}} \left(\frac{(4\pi * 10^(-7) \, \text{T m/A}) \cdot i}{2\pi \cdot r}\right) \cdot (0.12 \, \text{m} \, dx) \right) \]`

Complete question:

The current in the long, straight wire AB shown in the figure is upward and is increasing steadily at a rate di/dt.

(a) At an instant when the current is i, what are the magnitude and direction of the field B at a distance r to the right of the wire?

(b) What is the magnetic flux through the narrow, shaded strip?

(c) What is the total flux through the loop?

(d) What is the induced emf in the loop?

(e) Evaluate the numerical value of the induced emf if a = 12.0 cm, b = 36.0 cm, L = 24.0 cm, and di/dt = 9.60 A/s.