Question

A straight line has a gradient of 4 and passes though the point 2,11

work out the equation.
give your answer in the form y=mx+c where m and c are integers or fractions in their simplest form

Answer 1

y = 4x + 3. Get the y-intercept by subtracting 8.

Answer 2

Equation:

y = 4x + 3

Step-by-step explanation:

First, I am going to write the line's equation in point-slope:

`\sf{y-y_1=m(x-x_1)}`

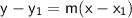
Where:

• m = slope
• y₁ is the y-coordinate of the point
• x₁ is the x-coordinate of the point

For this problem,

• m = 4
• y₁ = 11
• x₁ = 2

Next, I plug in the data:

`\sf{y-11=4(x-2)}`

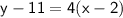
Then, I simplify to slope-intercept:

`\large\begin{gathered}\sf{y-11=4(x-2)}\\\sf{y-11=4x-8}\\\sf{y=4x-8+11}\\\sf{y=4x+3}\end{gathered}`

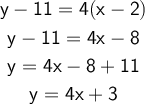
Hence, the equation in slope-intercept is y = 4x + 3.

`\rule{350}{4}`

`\frak{-Star-}`