Question

Three children are riding on the edge of a merry-go-round that is 182 kg, has a 1.60 m radius, and is spinning at 15.3 rpm. The children have masses of 17.4, 28.5, and 32.8 kg. If the child who has a mass of 28.5 kg moves to the center of the merry-go-round, what is the new angular velocity in rpm

Answer 1

The new angular velocity of the merry-go-round is 18.388 revolutions per minute.

Step-by-step explanation:

The merry-go-round can be represented by a solid disk, whereas the three children can be considered as particles. Since there is no external force acting on the system, we can apply the principle of angular momentum conservation:

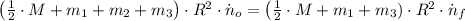`\left((1)/(2)\cdot M + m_(1)+m_(2) + m_(3) \right)\cdot R^(2)\cdot \dot n_(o) = \left((1)/(2)\cdot M + m_(1) + m_(3))\cdot R^(2)\cdot \dot n_(f)` (1)

Where:

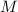`M` - Mass of the merry-go-round, in kilograms.

`m_(1)`,
`m_(2)`,
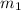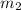`m_(3)` - Masses of the three children, in kilograms.

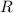`R` - Radius of the merry-go-round/Distance of the children with respect to the center of the merry-go-round, in meters.

`\dot n_(o)`,
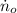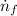`\dot n_(f)` - Initial and final angular speed, in revolutions per minute.

If we know that
`M = 182\,kg`,
`m_(1) = 17.4\,kg`,
`m_(2) = 28.5\,kg`,
`m_(3) = 32.8\,kg`,
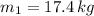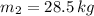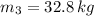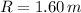`R = 1.60\,m` and
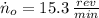`\dot n_(o) = 15.3\,(rev)/(min)`, then the final angular speed of the system is:

`\dot n_(f) = \dot n_(o)\cdot \left(((1)/(2)\cdot M + m_(1) + m_(2) + m_(3) )/((1)/(2)\cdot M + m_(1) + m_(3) ) \right)`

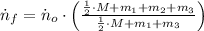
`\dot n_(f) = \left(15.3\,(rev)/(min) \right)\cdot \left[((1)/(2)\cdot (182\,kg) + 17.4\,kg +28.5\,kg + 32.8\,kg )/((1)/(2)\cdot (182\,kg) + 17.4\,kg + 32.8\,kg ) \right]`

`\dot n_(f) = 18.388\,(rev)/(min)`

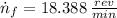
The new angular velocity of the merry-go-round is 18.388 revolutions per minute.