Question

Identify if it’s linear or quadratic

Answer 1

(A) -
`f(g(x))=-18x^2+27x-19`

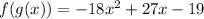
(B) - Quadratic

(C) - x=3/4

Explanation:

Given:

`f(x)=-2x^2+x-9\\\\g(x)=3x-2`

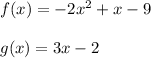
Find:

(A) -
`f(g(x))= \ ??`

(B) - Determine if f(g(x)) is linear or quadratic

(C) - Identify the slope or axis of symmetry

`\hrulefill`

Part (A) -

Simply plug the function g(x) into f(x) to find f(g(x)):

`f(g(x))=-2(3x-2)^2+(3x-2)-9`

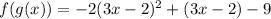
Simplifying:

`\therefore \boxed{f(g(x))=-18x^2+27x-19}`

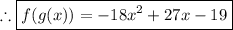
Thus, part (A) is solved.

Part (B) -

To determine if a function is linear or quadratic, you need to examine its form and characteristics. Here are some key differences between linear and quadratic functions:

Linear Function:

• The general form of a linear function is f(x) = mx + b, where m and b are constants.

• A linear function represents a straight line on a graph.

• The degree of a linear function is 1, meaning the highest power of the variable (x) is 1.

• In a linear function, the rate of change (slope) remains constant.

Quadratic Function:

• The general form of a quadratic function is f(x) = ax^2 + bx + c, where a, b, and c are constants, and a ≠ 0.

• A quadratic function represents a curve (parabola) on a graph.

• The degree of a quadratic function is 2, as the highest power of the variable (x) is 2.

• In a quadratic function, the rate of change (slope) is not constant and varies as x changes.

Using the information above we can determine f(g(x)) is quadratic.

Part (C) -

The axis of symmetry of a quadratic function can be found by using the formula x = -b / (2a), where a and b are the coefficients of the quadratic function in standard form. The resulting x-coordinate represents the vertical line that divides the parabola into two equal halves.

`\text{In our case}: \ a=-18 \ \text{and} \ b=27\\\\\\\Longrightarrow x=(-27)/(2(-18)) \\\\\\\therefore \boxed{x=(3)/(4) }`

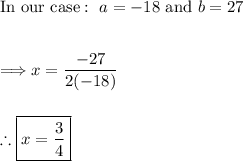
Thus, part (C) is solved.