Question

AB is a radius of the circle. The circumference of the circle is 119.56. Find the

length of AB, rounding to the nearest tenth.
B
A

Answer 1

To find the length of AB, we can use the formula for the circumference of a circle:

Circumference = 2 * π * radius

In this case, the given circumference is 119.56, and we are given that AB is a radius. Let's solve for AB:

119.56 = 2 * π * AB

Dividing both sides by 2π, we get:

AB = 119.56 / (2π)

Using the value of π as approximately 3.14, we can calculate the length of AB:

AB ≈ 119.56 / (2 * 3.14)
AB ≈ 19.05

Rounding to the nearest tenth, the length of AB is approximately 19.1. Therefore, the answer is 19.1.

Answer 2

19.0285

Explanation:

For this, we have to work backwards.

First, the equation for the circumference of a circle is:

`2\pi r`

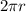
We already know the circumference, so we can solve for r:

`119.56=2\pi r`

divide both sides by 2

`59.78=\pi r`

divide both sides by pi

`19.0285...=r`

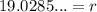
So, depending on the answer choices, the answer is 19.0285 rounded to the according decimal place.

Hope this helps! :)