Question

I just need the answer to this question please

Answer 1

`\begin{aligned} \textsf{(a)} \quad f(g(x))&=\boxed{x}\\g(f(x))&=\boxed{x}\end{aligned}\\\\\textsf{\;\;\;\;\;\;\;\;$f$ and $g$ are inverses of each other.}`

`\begin{aligned} \textsf{(b)} \quad f(g(x))&=\boxed{-x}\\g(f(x))&=\boxed{-x}\end{aligned}\\\\\textsf{\;\;\;\;\;\;\;\;$f$ and $g$ are NOT inverses of each other.}`

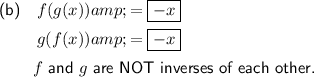
Explanation:

Part (a)

Given functions:

`\begin{cases}f(x)=x-2\\g(x)=x+2\end{cases}`

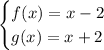
Evaluate the composite function f(g(x)):

`\begin{aligned}f(g(x))&=f(x+2)\\&=(x+2)-2\\&=x\end{aligned}`

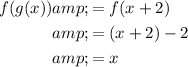
Evaluate the composite function g(f(x)):

`\begin{aligned}g(f(x))&=g(x-2)\\&=(x-2)+2\\&=x\end{aligned}`

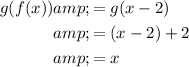
The definition of inverse functions states that two functions, f and g, are inverses of each other if and only if their compositions yield the identity function, i.e. f(g(x)) = g(f(x)) = x.

Therefore, as f(g(x)) = g(f(x)) = x, then f and g are inverses of each other.

`\hrulefill`

Part (b)

Given functions:

`\begin{cases}f(x)=(3)/(x),\;\;\;\:\:x\\eq0\\\\g(x)=-(3)/(x),\;\;x \\eq 0\end{cases}`

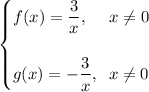
Evaluate the composite function f(g(x)):

`\begin{aligned}f(g(x))&=f\left(-(3)/(x)\right)\\\\&=(3)/(\left(-(3)/(x)\right))\\\\&=3 \cdot (-x)/(3)\\\\&=-x\end{aligned}`

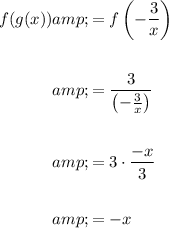
Evaluate the composite function g(f(x)):

`\begin{aligned}g(f(x))&=g\left((3)/(x)\right)\\\\&=-(3)/(\left((3)/(x)\right))\\\\&=-3 \cdot (x)/(3)\\\\&=-x\end{aligned}`

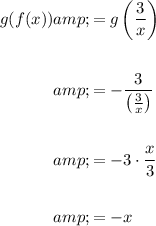
The definition of inverse functions states that two functions, f and g, are inverses of each other if and only if their compositions yield the identity function, i.e. f(g(x)) = g(f(x)) = x.

Therefore, as f(g(x)) = g(f(x)) = -x, then f and g are not inverses of each other.