Question

Find the equation of bisectors of the acute angle between the following pair of lines.

x+2y=5 and 4x+2y+9=0

Please help!​

Answer 1

The bisector of the acute angle is 6x + 6y - 1 = 0.

Explanation:

Let line x + 2y = 5 be defined by the equation a₁x + b₁y + c₁ = 0.

Let line 4x + 2y + 9 = 0 be defined by the equation a₂x + b₂y + c₂ = 0.

The formulas for the two angle bisectors of lines a₁x + b₁y + c₁ = 0 and a₂x + b₂y + c₂ = 0 are:

`\boxed{\frac{a_1x+b_1y+c_1}{\sqrt{{a_1}^2+{b_1}^2}}=\pm\frac{a_2x+b_2y+c_2}{\sqrt{{a_2}^2+{b_2}^2}}}`

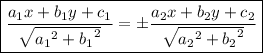
The two angle bisectors are perpendicular.

Substitute the values of a₁, b₁, c₁, a₂, b₂, and c₂ into both formulas.

Equation of bisector 1

`\begin{aligned}(x+2y-5)/(√(1^2+2^2))&=(4x+2y+9)/(√(4^2+2^2))\\\\(x+2y-5)/(√(5))&=(4x+2y+9)/(2√(5))\\\\x+2y-5&=(4x+2y+9)/(2)\\\\2(x+2y-5)&=4x+2y+9\\\\2x+4y-10&=4x+2y+9\\\\2x-2y+19&=0\end{aligned}`

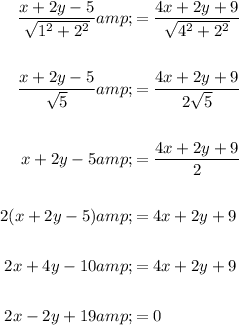
Equation of bisector 2

`\begin{aligned}(x+2y-5)/(√(1^2+2^2))&=-(4x+2y+9)/(√(4^2+2^2))\\\\(x+2y-5)/(√(5))&=-(4x+2y+9)/(2√(5))\\\\x+2y-5&=-(4x+2y+9)/(2)\\\\-2(x+2y-5)&=4x+2y+9\\\\-2x-4y+10&=4x+2y+9\\\\6x+6y-1&=0\end{aligned}`

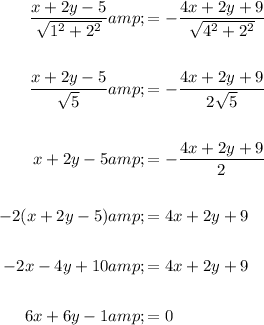
Therefore, the equations of bisectors of the angles between the given lines are:

`2x-2y+19=0`

`6x+6y-1=0`

`\hrulefill`

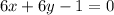
To identify the bisector of the acute angle, we need to calculate the angle between any one of the bisectors and one of the lines.

The formula for the angle between two lines a₁x + b₁y + c₁ = 0 and a₂x + b₂y + c₂ = 0 is:

`\tan \theta=\left|(a_2b_1-a_1b_2)/(a_1a_2+b_1b_2) \right|`

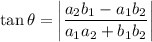
Let's find the angle θ between the bisector 6x + 6y - 1 = 0, and the line 4x + 2y + 9 = 0.

Therefore:

• a₁ = 6
• b₁ = 6
• a₂ = 4
• b₂ = 2

Substitute these values into the formula for the angle between two lines:

`\tan \theta=\left|((4)(6)-(6)(2))/((6)(4)+(6)(2)) \right|`

`\tan \theta=\left|(12)/(36) \right|`

`\tan \theta=\left|(1)/(3) \right|`

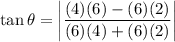
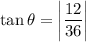
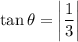
As tan θ < 1, the angle θ between the bisector and the line must be less than 45°. This means that the angle between the two given lines is less than 90°.

Acute angle measures less than 90°, so this means that 6x + 6y - 1 = 0 is the bisector of the acute angle between the given lines.

Note: On the attached diagram, the given lines are shown in black, the bisector of the acute angle is the red dashed line, and the bisector of the obtuse angle is the green dashed line.