Question

The relationship between women's whole-

number shoe sizes and foot length forms an
arithmetic sequence, where an is the foot
length in inches that corresponds to a shoe
size of n. A women's size 3 fits a foot 8
inches long and women's size 7 fits a foot
1
9 inches long.
What is the common difference of the arithmetic
sequence?
The common difference is
DONE
inch.

Answer 1

The common difference of the arithmetic sequence is 11/4 inches

Explanation:

The relationship between women's whole-number shoe sizes and foot length forms an arithmetic sequence

The formula for an arithmetic sequence is,

`a_n = a_1 + (n-1)d`

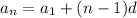
Now,

here, a_n is the foot length in inches that corresponds to a shoe size of n

now,

A women's size 3 fits a foot 8 inches long

Which give,

a_3 = a_1 + (3 - 1)d

but we are given that feet = 8 inches, so a_3 = 8.

so,

8 = a_1 + 2d (i),

or,

`8 = a_1 + 2d`

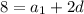
Also,

women's size 7 fits a foot 19 inches long.

So,

a_7 = 19 hence,

19 = a_1 + (7-1)d

19 = a_1 + 6d (ii)

or

`19 = a_1 + 6d`

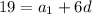
Using (i) and (ii) to find d,

from (i),

`8 = a_1 + 2d\\8 - 2d = a_1`

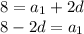
Putting value of a_1 into (ii)

19 = (8 - 2d) + 6d

19 = 8 - 2d + 6d

19 - 8 = 4d

11 = 4d

d = 11/4

Hence, the common difference is 11/4

Answer 2

first part: 1/3

second part: D. an= 22/3 +(n-1) 1/3

third part: 12 shoe

Explanation: