Question

Evaluate the following expression. Express your answer as a fraction or a decimal number rounded to four decimal places. 11 P 6/11 C 5 Must be rounded to four decimals

Answer 1

To evaluate the expression 11 P 6/11 C 5, calculate the value of the permutation 11P6 and the combination 11C5, and then divide the permutation by the combination.

Step-by-step explanation:

To evaluate the expression 11 P 6/11 C 5, we need to calculate the value of the permutation 11 P 6 (denoted as 11P6) and the combination 11 C 5 (denoted as 11C5), and then divide the result of the permutation by the result of the combination.

11P6 = 11! / (11-6)! = 11! / 5!

11C5 = 11! / (5!(11-5)!) = 11! / (5! * 6!)

Finally, we divide 11P6 by 11C5 to get the final result.

Answer 2

1. The value of
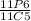`\( \frac{{11P6}}{{11C5}} \)` rounded to four decimal places is approximately 16.3636.

Step-by-step explanation:

The expression
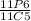`\( \frac{{11P6}}{{11C5}} \)`represents the permutation of 11 elements taken 6 at a time divided by the combination of 11 elements taken 5 at a time.

Permutation (nPm): Permutation refers to the arrangement of objects in a specific order. For this expression, 11P6 means selecting and arranging 6 elements out of 11 in a specific order. The formula for permutation is
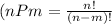`(nPm = \frac{{n!}}{{(n-m)!}}\)`, where n! denotes the factorial of n.

Combination (nCk):Combination, on the other hand, refers to the selection of objects without considering the order. For 11C5, it means choosing 5 elements out of 11 without any specific order. The combination formula is
`\(nCk = \frac{{n!}}{{k! \cdot (n-k)!}}\).`
`\( \frac{{11P6}}{{11C5}} \)`

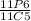
Calculating 11P6 and 11C5 separately and then finding their quotient yields the result, approximately 16.3636, rounded to four decimal places.

This explanation breaks down the expression into its permutation and combination components, providing a clear understanding of the mathematical operations involved in the calculation. It also emphasizes the distinction between permutation and combination.