Question

A rectangle has a length of (2.4±0.1)m and a width of (1.2±0.2)m, Calculate the area and the perimeter of the rectangle, and give the uncertainty in each value. (a) Calculate the area and give its uncertainty. (Enter your answers in m

2
.) सR m
2
±m (b) Calculate the perimeter of the rectangle and give its uncertainty. (Enter your answers in m.) बR m±m

Answer 1

(a) The area of the rectangle is
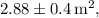`\(2.88 \pm 0.4 \, \text{m}^2\),` where the uncertainty is determined by propagating the uncertainties in the length and width.

(b) The perimeter of the rectangle is
`\(9.2 \pm 0.6 \, \text{m}\),` taking into account the uncertainties in both the length and width.

Step-by-step explanation:

(a) To calculate the area (A) of the rectangle, we use the formula
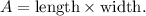`\(A = \text{length} * \text{width}\).`The uncertainties in the length (l) and width (w) contribute to the overall uncertainty in the area. The formula for the propagation of uncertainties in the product of two variables
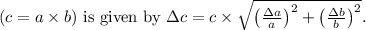`(\(c = a * b\)) is given by \(\Delta c = c * \sqrt{\left((\Delta a)/(a)\right)^2 + \left((\Delta b)/(b)\right)^2}\).` Applying this formula to the area, we find
`\(\Delta A = A * \sqrt{\left((\Delta l)/(l)\right)^2 + \left((\Delta w)/(w)\right)^2}\).`

(b) The perimeter (P) of a rectangle is given by the formula
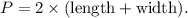`\(P = 2 * (\text{length} + \text{width})\).` Similarly, we account for uncertainties in both the length and width to determine the uncertainty in the perimeter using the same propagation formula.

For the given rectangle with a length of
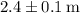`\(2.4 \pm 0.1 \, \text{m}\)` and a width of
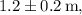`\(1.2 \pm 0.2 \, \text{m}\),` substitute these values into the formulas to obtain the final results.

Therefore, the area of the rectangle is
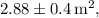`\(2.88 \pm 0.4 \, \text{m}^2\),`and the perimeter is
`\(9.2 \pm 0.6 \, \text{m}\),` considering the uncertainties in the measurements.