Answer: the answer will be (2,4)
Explanation:
Step 1: Solve y=3x−2 for y:
y=3x-2
Step 2: Substitute 3x−2 for y in y−2=x:
y−2=x
3x−2−2=x
3x−4=x (Simplify both sides of the equation)
3x−4+−x=x+−x (Add -x to both sides)
2x−4=0
2x−4+4=0+4 (Add 4 to both sides)
2x=4
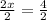 (divide both sides by 2)
(divide both sides by 2)
x=2
Step 3: Substitute 2 for x in y=3x−2:
y=3x-2
y=(3)(2)-2
y=4 (Simplify both sides of the equation)
Answer:
x=2 , y=4