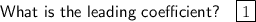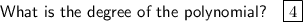Question

Please help I am so lost and confused thank you all

Answer 1

`\begin{array}{lcc}& \sf degree=& \sf coefficient=\\\sf First\;term:&\boxed{0}&\boxed{3}\\\\& \sf degree=& \sf coefficient=\\\sf Second\;term:&\boxed{3}&\boxed{-1}\\\\& \sf degree=& \sf coefficient=\\\sf Third\;term:&\boxed{4}&\boxed{1}\\\\& \sf degree=& \sf coefficient=\\\sf Fourth\;term:&\boxed{1}&\boxed{5}\\\\& \sf degree=& \sf coefficient=\\\sf Fifth\;term:&\boxed{2}&\boxed{2}\\\\\end{aligned}`

`\textsf{What is the leading coefficient?\;\; $\boxed{1}$}`

`\textsf{What is the degree of the leading term?\;\; $\boxed{4}$}`

`\textsf{What is the degree of the polynomial?\;\; $\boxed{4}$}`

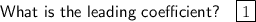
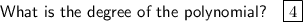
Explanation:

In a polynomial expression, the degree of a term refers to the exponent of the variable of that term. If there is no variable, the degree is zero.

In a polynomial expression, the coefficient of a term refers to the numerical value that appears in front of the variable of that term. If there is no coefficient, the coefficient is 1.

Given polynomial:

`3-x^3+x^4+5x+2x^2`

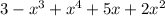
Note that the terms of the given polynomial are not in any specific order.

We have not been asked to order them, therefore the first term is 3, the second term is -x³, and so on.

Therefore:

`\begin{array}{lcc}& \sf degree=& \sf coefficient=\\\sf First\;term:&\boxed{0}&\boxed{3}\\\\& \sf degree=& \sf coefficient=\\\sf Second\;term:&\boxed{3}&\boxed{-1}\\\\& \sf degree=& \sf coefficient=\\\sf Third\;term:&\boxed{4}&\boxed{1}\\\\& \sf degree=& \sf coefficient=\\\sf Fourth\;term:&\boxed{1}&\boxed{5}\\\\& \sf degree=& \sf coefficient=\\\sf Fifth\;term:&\boxed{2}&\boxed{2}\\\\\end{aligned}`

In a polynomial expression, the leading term refers to the term with the highest degree. Therefore, the leading term of the given polynomial is x⁴.

The leading coefficient refers to the coefficient of the leading term.

The leading term is x⁴, so the leading coefficient is 1.

The degree of the leading term of a polynomial refers to the exponent of the leading term. The leading term is x⁴, so the degree of the leading team is 4.

The degree of a polynomial is the exponent of the leading term. The leading term is x⁴, so the degree of the polynomial is 4.

`\textsf{What is the leading coefficient?\;\; $\boxed{1}$}`

`\textsf{What is the degree of the leading term?\;\; $\boxed{4}$}`

`\textsf{What is the degree of the polynomial?\;\; $\boxed{4}$}`