Question

Can someone help me please with these problems?

Answer 1

(7) - Option (1)

(8) - Option (4)

Refer to the step-by-step.

Explanation:

(7) - Rewrite the following expression as fractional exponent.

`(√(x) \cdot x)/(x^(-2))`

We can use the rules for exponents to solve this problem.

`\boxed{\left\begin{array}{ccc}\text{\underline{Exponent rules:}}\\\\1.\ a^0=1\\\\2.\ a^m * a^n=a^(m+n)\\\\3.\ a^m / a^n=a^(m-n)\\\\4.\ (ab)^m=a^mb^m\\\\5.\ (a/b)^m=a^m/b^m\\\\6.\ (a^m)^n=a^(mn)\\\\7.\ a^(-m)=1/a^m\\\\8.\ a^(m/n)=(\sqrt[n]{a} )^m\end{array}\right}`

`(√(x) \cdot x)/(x^(-2))\\\\\\\Longrightarrow \frac{x^{(1)/(2) } \cdot x}{x^(-2)} \ \text{(Used rule 8)} \\\\\\\Longrightarrow \frac{x^{(1)/(2)+1 } }{x^(-2)}\\\\\\\Longrightarrow \frac{x^{(3)/(2) } }{x^(-2)} \ \text{(Used rule 2)}\\\\\\ \Longrightarrow x^{(3)/(2)-(-2) } \\ \\ \\\Longrightarrow \boxed{x^{(7)/(2) }} \ \text{(Used rule 3)}`

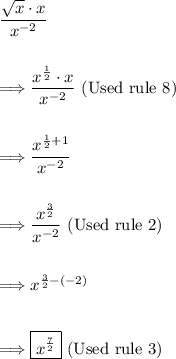
Thus, option (1) is correct.

`\hrulefill`

(8) - Express the following expression as a radical

`\frac{x^{(1)/(3) }x^{(1)/(2) }}{x^(-1)}`

Once again, we can use the rules for exponents to solve this problem.

`\frac{x^{(1)/(3) }x^{(1)/(2) }}{x^(-1)}\\\\\\\Longrightarrow \frac{x^{(1)/(3) +(1)/(2) }}{x^(-1)} \\\\\\\Longrightarrow \frac{x^{(5)/(6) }}{x^(-1)} \ \text{(Used rule 2)} \\ \\ \\ \Longrightarrow x^{(5)/(6)-(-1) }\\\\\\\Longrightarrow x^{(11)/(6) }} \ \text{(Used rule 3)} \\ \\ \\ \Longrightarrow \boxed{\sqrt[6]{x^(11)}} \ \text{(Used rule 8)}`

Thus, option (4) is correct.