Question

Look over Chuck's work What is incorrect about the way Chuck interpreted his problem? What should have been a clue to Chuck that something was wrong?

Answer 1

See below

Explanation:

He misinterpreted P(A|B) as P(A∩B) and calculated that instead. P(A|B)=0.17 represents the probability that the student takes Chemistry given they've taken Algebra 2, while P(A∩B) represents the probability that the student takes both Chemistry and Algebra 2. Here's how the problem should've been done:

`\displaystyle P(A|B)=(P(A\cap B))/(P(B))\\\\0.17=(P(A\cap B))/(0.8)\\\\0.136=P(A\cap B)`

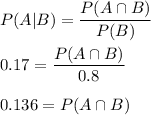
Therefore, the correct probability that the student will take both Chemistry and Algebra 2 is 0.136, or 13.6%