Question

Determine the equation of the ellipse with foci... Please put the dots on the graph thats in the image. 100 points

Answer 1

`((x-7)^2)/(8^2) + ((y-2)^2)/(17^2) = 1`

Explanation:

Major axis length 2a

⇒ 2a = 34

⇒ a = 34/2

⇒ a = 17

General eq of ellipse:

`((x-h)^2)/(b^2) + ((y-k)^2)/(a^2) = 1`

centre : (h,k)

foci: (h, k+c) and (h,k-c)

Gn. foci : (7, 17) and (7, -13)

Comparing the above 2 lines,

h = 7,

k + c = 17 -eq(1)

k - c = -13 -eq(2)

eq(1) + eq(2):

(k + c) + (k - c) = 17 + (-13)

2k = 4

k = 2

sun k = 2 in eq(1):

2 + c = 17

c = 15

Also, c² = a² - b²

b² = a² - c²

= 17² - 15²

= 64

b² = 8²

b = 8

substituting in ellipse eq,

`((x-7)^2)/(8^2) + ((y-2)^2)/(17^2) = 1`

Answer 2

`((x-7)^2)/(64)+((y-2)^2)/(289)=1`

Explanation:

As the foci of the ellipse have the same x-value, the ellipse is vertical.

The formula for a vertical ellipse is:

`\boxed{((x-h)^2)/(a^2)+((y-k)^2)/(b^2)=1}`

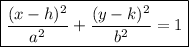
where:

• b > a
• b is the major radius and 2b is the major axis.
• a is the minor radius and 2a is the minor axis.
• Center = (h, k)
• Vertices = (h, k±b)
• Co-vertices = (h±a, k)
• Foci = (h, k±c) where c² = b² - a²

Given the major axis is 34:

• 2b = 34
• b = 17
• b² = 289

The center of an ellipse is located at the midpoint between its two foci.

Given the foci are (7, 17) and (7, -13), the center of the ellipse is:

`(h, k) = (7, 2)`

As the formula for the foci is (h, k±c), then (k, h±c) = (7, 2±c). Therefore:

`\begin{aligned}2\pm c &= 17, -13\\\pm c &= 15, -15\\c &= 15\end{aligned}`

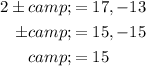
The vertices are:

`\begin{aligned}(h, k\pm b) &= (7, 2 \pm 17)\\& = (7, 19) \; \textsf{and}\; (7, -15)\end{aligned}`

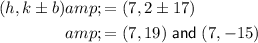
To find the value of a, substitute the values of b and c into c² = b² - a²:

`\begin{aligned}c^2&=b^2-a^2\\15^2&=17^2-a^2\\a^2&=√(17^2-15^2)\\a^2&=64\\a&=8\end{aligned}`

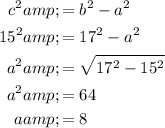
The co-vertices are:

`\begin{aligned}(h \pm a, k) &= (7 \pm 8, 2)\\& = (-1,2) \; \textsf{and}\; (15,2)\end{aligned}`

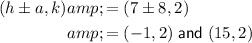
Therefore:

• a = 8 ⇒ a² = 64
• b = 17 ⇒ b² = 289
• h = 7
• k = 2

To find the equation of the ellipse, substitute these values into the formula:

`\boxed{((x-7)^2)/(64)+((y-2)^2)/(289)=1}`

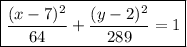
• Major axis, 2b = 34
• Minors axis, 2a = 16
• Center = (7, 2)
• Vertices = (7, -15) and (7, 19)
• Co-vertices = (-1, 2) and (15, 2)
• Foci = (7, 17) and (7, -13)