Question

Find the volume of the cone in cubic feet. Use 3.14 for π and round your answer to the nearest tenth.

ANSWER OPTIONS
(1 m ≈ 3.2808 ft)
A. 3,783.9 ft3
B. 5,083,662.2 ft3
C. 104,705.4 ft3
D. 143,958.5 ft3

Answer 1

B. 5,083,662.2 ft³

Explanation:

The question asks us to calculate the volume of a cone in cubic feet, but it has provided the measurements of height and radius in metres.

Therefore, we have to first convert the given measurements into units of feet using the provided conversion factor:

• height = 65 m

= 65 m × 3.2808 ft/m

= 213.252 ft

• radius = 46 m

= 46 m × 3.2808 ft/m

= 150.9168 ft

Now that we have the measurements in the required units, we have to calculate the volume of the cone.

The formula for the volume of a right-circular cone is:

`\boxed{V = (1)/(3)\pi r^2 h}`,

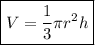where:

V = volume of the cone

r = radius of the circular base

h = perpendicular height of the cone

Substituting the required values into the formula above, we get:

`V = (1)/(3) * 3.14 * (150.9168)^2 * 213.252`

`= 5083662.17`

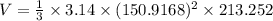
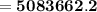`= \bf 5083662.2` [Rounding to the nearest tenth]

Therefore, the correct option is B.