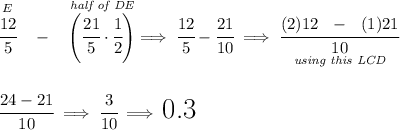well, if we take a peek at the number line above, from one integer to the next there are 5 slots or divisions, meaning each division is 1/5.
what's the distance of DE?
well, D is at -1 plus 4 slots, that means -1 and we move it further by 4/5, that makes it -1⅘.
now E is at 2 plus 2 slots more, so that means 2 and we move it further by 2/5 or 2⅖.
to get the distance we simply get their difference, not much to it.
![\stackrel{mixed}{1(4)/(5)}\implies \cfrac{1\cdot 5+4}{5}\implies \stackrel{improper}{\cfrac{9}{5}}~\hfill \stackrel{mixed}{2(2)/(5)} \implies \cfrac{2\cdot 5+2}{5} \implies \stackrel{improper}{\cfrac{12}{5}} \\\\[-0.35em] ~\dotfill\\\\ |DE|\implies \left| -\cfrac{9}{5}-\cfrac{12}{5} \right|\implies \left| -\cfrac{21}{5} \right|\implies \cfrac{21}{5}\implies \text{\LARGE 4.2}](https://img.qammunity.org/2024/formulas/mathematics/college/ww57oezur3oykyxzes9xk4ii64onm29x9t.png)
how about the midpoint of DE?
well, we can move from the left over to the midpoint or we can move from the right, let's move from the right, so hmmm let's take half of 21/5 and subtract it from E, that's where the midpoint is.