Question

The graph of a function h (x) is shown.

What is the average rate of change of h(x) over the interval [4, 8]?
A)-6
B)-2
C)-32
D)-23

Answer 1

`\textsf{C)} \quad -(3)/(2)`

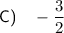
Explanation:

To find the average rate of change of a function over an interval, we can use the formula:

`\boxed{\begin{minipage}{6.3 cm}\underline{Average rate of change of function $f(x)$}\\\\$(f(b)-f(a))/(b-a)$\\\\over the interval $a \leq x \leq b$\\\end{minipage}}`

In this case, the interval is [4, 8], so:

• a = 4
• b = 8

From inspection of the given graph:

• h(a) = h(4) = 9
• h(b) = h(8) = 3

Substitute the values into the formula to calculate the average rate of change:

`\begin{aligned}\text{Average rate of change}&=(h(8)-h(4))/(8-4)\\\\&=(3-9)/(8-4)\\\\&=(-6)/(4)\\\\&=-(3)/(2)\end{aligned}`

Therefore, the average rate of change of h(x) over the interval [4, 8] is -3/2.