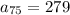Question

Find the 75th term of the arithmetic sequence -17, -13, -9....

Answer 1

The 75th term of the arithmetic sequence -17, -13, -9.... is:

`a_(75)=279`

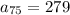
Explanation:

Given the sequence

`-17, -13, -9....`

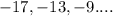
An arithmetic sequence has a constant difference 'd' and is defined by

`a_n=a_1+\left(n-1\right)d`

computing the differences of all the adjacent terms

`-13-\left(-17\right)=4,\:\quad \:-9-\left(-13\right)=4`

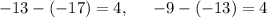
The difference between all the adjacent terms is the same and equal to

`d=4`

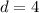
The first element of the sequence is:

`a_1=-17`

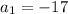
now substitute
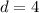`d=4` and
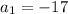`a_1=-17` in the nth term of the sequence

`a_n=a_1+\left(n-1\right)d`

`a_n=4\left(n-1\right)-17`

`a_n=4n-21`

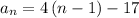
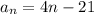
Now, substitute n = 75 in the
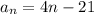`a_n=4n-21` sequence to determine the 75th sequence

`a_n=4n-21`

`a_(75)=4\left(75\right)-21`

`a_(75)=300-21`

`a_(75)=279`

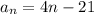
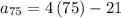
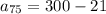
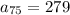
Therefore, the 75th term of the arithmetic sequence -17, -13, -9.... is:

`a_(75)=279`