Final answer
∠B1 = 30.0 degrees
∠B2 = 150.0 degrees
Explanation
To find the two possible angles
 between
between
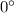 and
and
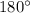 with the given value for sine, follow these steps. First, consider the sine function's periodicity, which occurs every
with the given value for sine, follow these steps. First, consider the sine function's periodicity, which occurs every
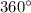 . Hence, for a given sine value, two angles exist within the range of
. Hence, for a given sine value, two angles exist within the range of
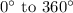 that satisfy the condition.
that satisfy the condition.
To determine the angles, begin by finding the reference angle using the inverse sine function. In this case, the reference angle is
 . As sine is positive in both the first and second quadrants, the acute angle
. As sine is positive in both the first and second quadrants, the acute angle
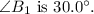 The second angle
The second angle
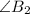 in the second quadrant can be calculated by subtracting the reference angle from
in the second quadrant can be calculated by subtracting the reference angle from
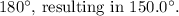
Therefore, the two angles that satisfy the conditions for the given sine value are
 (the acute angle) and
(the acute angle) and
 in the second quadrant.
in the second quadrant.