Question

Select the correct answer.
Which equation represents a line that is perpendicular to line FG?

Answer 1

y = -2x - 3

Explanation:

Two lines that are perpendicular to one another share the attribute that the product of their slopes is equal to -1.

Therefore, to find the line perpendicular to FG's slope, we'll find FG's slope and then use the said attribute.

We're given two points: G(9, 8) and F(-5, 1).

`m = (y_2 - y_1)/(x_2 - x_1)\\\\m_(FG) = (y_G - y_F)/(x_G - x_F) = (8 - 1)/(9 - (-5)) = \frac7{14} = \frac12`

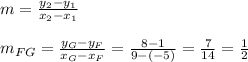
Using the attribute mentioned above, we know the following:

`m_(FG) \cdot m_(\perp FG) = -1\\\frac12 \cdot m_(\perp FG) = -1\\m_(\perp FG) = -2`

We don't know where this line intercepts FG, therefore, we can't find its equation. However, the answers only show one line with a slope of -2, therefore the equation of the line perpendicular to FG is y = -2x - 3.