Question

2

Scooter is saving money to build a race car while attending school. He receives
a small investment of $500,000 from Junebug (Dale Earnhardt Jr) to finance
this. Scooter puts this away in an account while he finishes school. The account
he chooses yields 9.95% annual interest compounded continuously.
Approximately how much money will Scooter have in his account after 7 years?

Answer 1

To calculate the amount of money Scooter will have in his account after 7 years with continuous compounding, we can use the formula for continuous compound interest:

`\sf\:A = P \cdot e^(rt) \\`

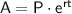
Where:

• A is the final amount of money in the account
• P is the initial principal (the investment amount)
• e is Euler's number (approximately 2.71828)
• r is the annual interest rate (in decimal form)
• t is the time period in years

Given:

• P = $500,000
• r = 9.95% (0.0995 in decimal form)
• t = 7 years

Substituting the values into the formula, we have:

`\sf\:A = 500,000 \cdot e^(0.0995 \cdot 7) \\`

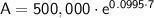
Calculating the exponential part:

`\sf\:A = 500,000 \cdot e^(0.6965) \\`

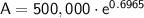
Using a calculator or mathematical software, we can find that
`\sf\:e^(0.6965) \approx 2.006 \\`.

Thus, the amount of money Scooter will have in his account after 7 years, with continuous compounding, is:

`\sf\:A \approx 500,000 \cdot 2.006 \approx \$1,003,000 \\`

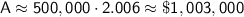
Therefore, approximately $1,003,000 will be in Scooter's account after 7 years.