Answer:
Rational as it is a terminating decimal.
Explanation:
Given expression:
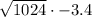
Rewrite 1024 as 32²:
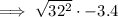
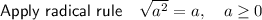

Multiply the numbers:

Therefore, the product is a terminating decimal.
A rational number is a number that can be expressed as the ratio of two integers (where the denominator does not equal zero).
A terminating decimal can be written as a rational number.
To convert a terminating decimal into a rational number, multiply the number by a multiple of 10 that eliminates the decimal, then divide by the same number:
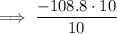
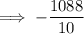
Reduce the fraction to its simplest form by dividing the numerator and denominator by 2:
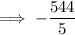
Therefore, the product of √1024 and -3.4 is rational as it is a terminating decimal.