Question

What is the surface area of the triangular prism???

Answer 1

S.A. = 36 ft²

Explanation:

The surface area of the given triangular prism is made up of two congruent triangles and three rectangles.

The dimensions of the triangles are:

• base, b = 3 ft
• height, h = 4 ft

The dimensions of the three rectangles are:

• Rectangle 1: 4 ft × 2 ft
• Rectangle 2: 3 ft × 2 ft
• Rectangle 3: 5 ft × 2 ft

The area of a triangle can be found by halving the product of its base and height. The area of a rectangle is the product of its width and length.

Therefore, the total surface area of the triangular prism is:

`\begin{aligned}\textsf{Surface area}&=\sf 2\;triangles+rectange\;1+rectangle\;2+rectangle\;3\\\\&=2 \left((1)/(2) \cdot 3 \cdot 4\right)+(4 \cdot 2)+(3 \cdot 2)+(5 \cdot 2)\\\\&=2(6)+8+6+10\\\\&=12+8+6+10\\\\&=20+6+10\\\\&=26+10\\\\&=36\; \sf ft^2\end{aligned}`

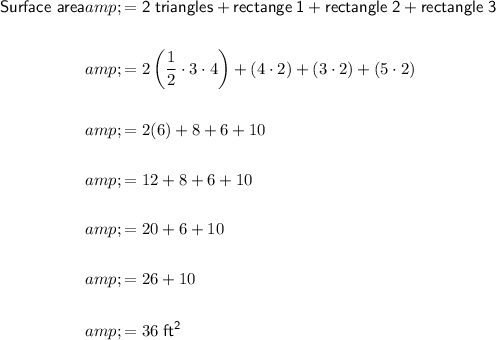
Therefore, the total surface area of the given triangular prism is 36 ft².

Answer 2

36ft^2

Explanation:

The formula for the surface area of a triangular prism is:

Surface area = (Perimeter of the base *Length of the prism) + (2*Base Area)

= (S1 +S2 + S3)L + 2*1/2*b*h

= (S1 +S2 + S3)L + b*h

where,

• b is the bottom edge of the base triangle,
• h is the height of the base triangle,
• L is the length of the prism and
• S1, S2, and S3 are the three edges (sides) of the base triangle
• (bh) is the combined area of the two triangular faces
  [2 × (1/2 × bh)] = bh

For Question:

S1= 4ft

S2= 3ft

S3= 5ft

L= 2ft

b= 3 ft

h= 4ft

Now

Surface area = (S1 +S2 + S3)L + bh

=(4+3+5)*2+3*4

=36 ft^2

Therefore the surface area of the triangular prism is 36ft^2