Answer:
Either
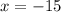 or
or
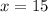 .
.
Explanation:
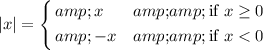 .
.
Hence, there are two possibilities to consider:
- Either
 ,
, - Or
 .
.
If
 , then
, then
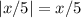 .
.
Substitute into the original equation:
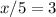 . Solve for
. Solve for
 :
:
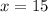 .
.
Important: verify that the solution
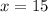 meet the assumption
meet the assumption
 . Indeed,
. Indeed,
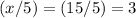 and is indeed non-negative. Hence, the solution
and is indeed non-negative. Hence, the solution
 is valid.
is valid.
On the other hand, if
 , then
, then
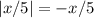 .
.
Substitute into the original equation:
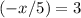 . Solve for
. Solve for
 :
:
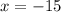 .
.
Similarly, verify that the solution
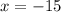 satisfies the current assumption that
satisfies the current assumption that
 . Indeed, this assumption is met, and
. Indeed, this assumption is met, and
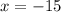 is also a valid solution.
is also a valid solution.