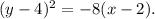Question

100 Points! Geometry question. Photo attached. Write the equation of the parabola with the given conditions. Please show as much work as possible. Thank you!

Answer 1

`(y - 4)^2 = -8(x - 2).`

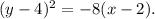
Explanation:

The equation of a parabola with a vertical axis of symmetry, vertex (h, k), and focus (h+a, k) is given by:

`(y - k)^2 = 4a(x - h)`

In this case, the vertex is (2, 4) and the focus is (0, 4).

Comparing this to the general equation, we have h=2, k=4, and h+a=0.

From h+a=0, we can solve for a:

a=-h = -2

Substituting the values of h, k, and p into the equation, we get:

`(y - 4)^2 = 4(-2)(x - 2)`

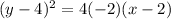
Simplifying further:

`(y - 4)^2 = -8(x - 2)`

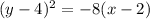
Therefore, the parabola equation is
`(y - 4)^2 = -8(x - 2).`