Question

NO LINKS!! URGENT HELP PLEASE!!!

Find the area of each regular polygon. Round your answer to the nearest tenth.

Answer 1

1. 779.42 unit^2

2. 585 unit^2

Explanation:

1.

no. of side (n)=6

apothem(a)=15

Each central angle=360/n =360/6=60 degree

Here angle AOZ =60/2=30 degree

In triangle AOZ with respect to O Tan O= opposite /base

Tan 30=AZ/15

AZ-Tan 30*15=
`5√(3)`

AB=
`2*5 √(3)=10 √(3)`

Therefore, the length of each side (s)=
`10 √(3)`

Now

perimeter(p)=n*s=
`6*10 √(3)=60 √(3)`Area

`Area=(P*a)/(2)`

substituting value:

`Area=(60√(3)*15)/(2)=\bold{779.42\: unit^2}`

`\hrulefill`

2.

no. of side (n)=6

length of one side(s)=15

Perimeter(p)=n*s=6*15=90 units

Now finding apothem(a),

`\bold{apothem(a)=(s)/(2Tan((180^o)/(n)))}`

by substituting value, we get,

`\bold{apothem(a)=(15)/(2Tan((180^o)/(6)))=12.99=13 units}`

Now, we have

`Area=(P*a)/(2)`

substituting value:

`Area=(90*13)/(2)=\bold{585\: unit^2}`

`\hrulefill`

Answer 2

1) 779.4 square units (nearest tenth)

2) 584.6 square units (nearest tenth)

Explanation:

To find the areas of the given regular polygons, first determine their side lengths and apothems, then use the area formula:

`\boxed{A=(n\cdot s\cdot a)/(2)}`

Question 1

The given diagram shows a six-sided regular polygon with an apothem measuring 15 units. Therefore:

• Number of sides: n = 6
• Apothem: a = 15

The formula for the apothem of a regular polygon is:

`\boxed{\begin{minipage}{5.5cm}\underline{Apothem of a regular polygon}\\\\$a=(s)/(2 \tan\left((180^(\circ))/(n)\right))$\\\\where:\\\phantom{ww}$\bullet$ $s$ is the side length.\\ \phantom{ww}$\bullet$ $n$ is the number of sides.\\\end{minipage}}`

Therefore, to find the side length, s, of the given regular polygon, substitute the values of a and n into the apothem formula and solve for s:

`\implies 15=(s)/(2 \tan\left((180^(\circ))/(6)\right))`

`\implies 15=(s)/(2 \tan\left(30^(\circ)\right))`

`\implies s=30\tan\left(30^(\circ)\right)`

`\implies s=30\cdot (√(3))/(3)`

`\implies s=10√(3)`

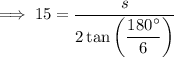
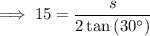
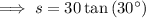
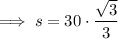
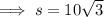
Therefore, the side length of the polygon is 10√3 units.

The formula for the area of a regular polygon is:

`\boxed{\begin{minipage}{6cm}\underline{Area of a regular polygon}\\\\$A=(n\cdot s\cdot a)/(2)$\\\\where:\\\phantom{ww}$\bullet$ $n$ is the number of sides.\\ \phantom{ww}$\bullet$ $s$ is the length of one side.\\ \phantom{ww}$\bullet$ $a$ is the apothem.\\\end{minipage}}`

Therefore, to find the area of the given regular polygon, substitute the values of n, s and a into the area formula and solve for A:

`\implies A=(6 \cdot 10√(3) \cdot 15)/(2)`

`\implies A=(900√(3))/(2)`

`\implies A=450√(3)`

`\implies A=779.4\; \sf square \; units\;(nearest\;tenth)`

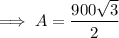
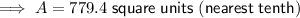
Therefore, the area of the given regular polygon is 779.4 square units (nearest tenth).

`\hrulefill`

Question 2

The given diagram shows a six-sided regular polygon with a side length measuring 15 units. Therefore:

• Number of sides: n = 6
• Side length: s = 15

The formula for the apothem of a regular polygon is:

`\boxed{\begin{minipage}{5.5cm}\underline{Apothem of a regular polygon}\\\\$a=(s)/(2 \tan\left((180^(\circ))/(n)\right))$\\\\where:\\\phantom{ww}$\bullet$ $s$ is the side length.\\ \phantom{ww}$\bullet$ $n$ is the number of sides.\\\end{minipage}}`

Therefore, to find the apothem, a, of the given regular polygon, substitute the values of s and n into the apothem formula and solve for a:

`\implies a=(15)/(2 \tan\left((180^(\circ))/(6)\right))`

`\implies a=(15)/(2 \tan\left(30^(\circ)\right))`

`\implies a=(15)/(2 \cdot (√(3))/(3))`

`\implies a=(15√(3))/(2)`

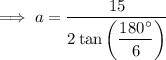
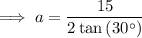
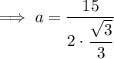
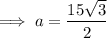
Therefore, the apothem of the polygon is (15√3)/2 units.

The formula for the area of a regular polygon is:

`\boxed{\begin{minipage}{6cm}\underline{Area of a regular polygon}\\\\$A=(n\cdot s\cdot a)/(2)$\\\\where:\\\phantom{ww}$\bullet$ $n$ is the number of sides.\\ \phantom{ww}$\bullet$ $s$ is the length of one side.\\ \phantom{ww}$\bullet$ $a$ is the apothem.\\\end{minipage}}`

Therefore, to find the area of the given regular polygon, substitute the values of n, s and a into the area formula and solve for A:

`\implies A=(6 \cdot 15 \cdot (15√(3))/(2))/(2)`

`\implies A=(675√(3))/(2)`

`\implies A=584.6\; \sf square \; units\;(nearest\;tenth)`

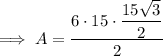
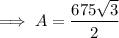
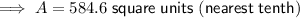
Therefore, the area of the given regular polygon is 584.6 square units (nearest tenth).