Answer:
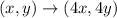which is choice (3)
======================================================
Step-by-step explanation:
The general transformation of
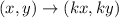describes a dilation with scale factor k. If k is not equal to 1, then the resulting image will not be the same size as the preimage.
In this case, k = 4. This means that the resulting image has its side lengths 4 times greater compared to the preimage side lengths. For example, if a side length is 5 units long in the preimage, then its corresponding length in the image is 5*4 = 20 units long.
If this is enlargement applied to all sides, then the perimeter also goes up by the same scale factor (since the perimeter is the sum of all exterior sides). The image perimeter will be four times that of the preimage perimeter.
In summary, if you apply a dilation (where k is not equal to 1), then the perimeter will change. If k > 1, then the perimeter gets larger. If 0 < k < 1, then the perimeter will shrink.
----------------------
Extra info:
- Choice (1) describes a reflection over the line y = x
- Choice (2) is a reflection over the x axis.
- Choice (4) describes a translation of 2 units to the right and 5 units down.
- The transformations of reflections, translations, and rotations all are considered rigid transformations. This means that they keep the same size and shape. You can imagine the camera is moving around in some way and the shapes stay still (but give the illusion of movement). In other words, rigid transformations will keep the perimeter the same. Because of this, we can rule out choices (1), (2) and (4).