Question

A line has a slope of –3 and passes through the point (6,–18). Write its equation in slope-intercept form. Write your answer using integers, proper fractions, and improper fractions in simplest form.

Answer 1

-3x

Explanation:

Let's plug the values into point-slope first. We have all the necessary info to perform such an operation - we have the point and the slope.

Point-slope is:

`\bf{y-y_1=m(x-x_1)}`

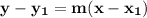
Where y₁ and x₁ are the co-ordinates of the point and m is the slope.

So we plug the data in, like this:

`\bf{y-(-18)=-3(x-6)}`

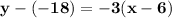
Now we can simplify

`\bf{y+18=-3(x-6)}`

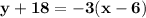
Use the distributive property

`\bf{y+18=-3x+18`

Subtract 18 from each side

`\bf{y=-3x}`

Therefore, the equation is -3x.

Answer 2

-18 = -3(6) + b

-18 = -18 + b

b = 0

y = -3x