Question

the top of an electric pole is s supported by a wire of 26 ft long on the ground level. how far is tightened spot from the foot of the pole if its height is 24 ft?​

Answer 1

The tightened spot is 10 feet away from the foot of the pole.

Explanation:

1. Draw the diagram. Notice that the shape of the electric pole and its supporting wire creates a right triangle.

2. We know 2 side lengths already (26ft, 24ft), and we need to find 1 more side length. Therefore, to find the 3rd side length of a right-triangle, utilize Pythagoras' Theorem.

⭐What is the Pythagoras' Theorem?

1. 
   `(C)^2 = (A)^2 + (B)^2`
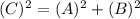
2. An equation to find a 3rd side length
3. C = hypotenuse
4. A = one leg
5. B = another leg

3. Substitute the values of the side lengths into the equation, and solve for the unknown side length.

Let B= the distance from the tightened spot to the foot of the pole.

`(C)^2 = (A)^2 + (B)^2`

`26^2 = 24^2 + B^2`

`676 = 576 + B^2`

`100 = B^2`

`√(100) = √((B)^2)`

`10 = B`

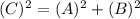
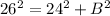
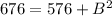
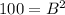
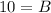
∴ The tightened spot is 10 feet away from the foot of the pole.

Diagram: