Question

NO LINKS!! URGENT HELP PLEASE!!!

43. Miles invested $2400 into a retirement account that earns 1.8% interest compounded bimonthly. Write a function to model this situation, then find when Miles will have $10,000 in the account?

44. Sarah moved $30,000 of her savings to a new investment account that earns 4% interest compounded quarterly. Write a function to model this situation, then find how many years until her values doubles?

Answer 1

43) 79 years and 6 months

44) 17 years and 6 months

Explanation:

As the account increases by a constant percentage bi-monthly, we can use the compound interest formula to write a function to model the situation.

`\boxed{\begin{minipage}{8.5 cm}\underline{Compound Interest Formula}\\\\$ A=P\left(1+(r)/(n)\right)^(nt)$\\\\where:\\\\ \phantom{ww}$\bullet$ $A =$ final amount \\ \phantom{ww}$\bullet$ $P =$ principal amount \\ \phantom{ww}$\bullet$ $r =$ interest rate (in decimal form) \\ \phantom{ww}$\bullet$ $n =$ number of times interest is applied per year \\ \phantom{ww}$\bullet$ $t =$ time (in years) \\ \end{minipage}}`

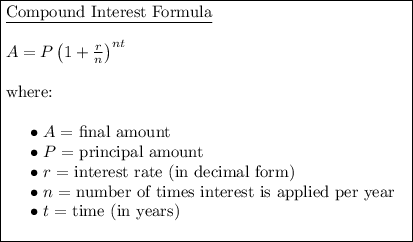
The interest is compounded bi-monthly, which means every 2 months.

Therefore, the interest is applied 6 times per year.

Given values:

• A = $10,000
• P = $2,400
• r = 1.8% = 0.018
• n = 6 (bi-monthly)

Substitute the given values into the formula, and solve for t:

`\begin{aligned}A&=P\left(1+(r)/(n)\right)^(nt)\\\\\implies 10000&=2400\left(1+(0.018)/(6)\right)^(6t)\\\\\implies 10000&=2400\left(1+0.003\right)^(6t)\\\\(25)/(6)&=\left(1.003\right)^(6t)\\\\\textsf{Take natural logs:} \quad \ln \left((25)/(6)\right)&=\ln\left(1.003\right)^(6t)\\\\\ln \left((25)/(6)\right)&=6t\ln\left(1.003\right)\\t&=(\ln \left((25)/(6)\right))/(6\ln\left(1.003\right))\\\\t&=79.4031089...\end{aligned}`

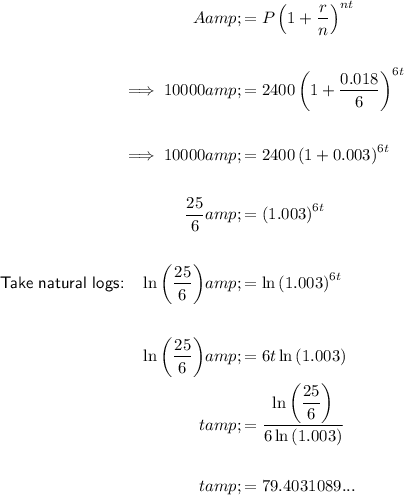
The account balance will reach $10,000 during the 79th year (after 79 years and 4.84 months).

As the interest is applied bi-monthly (every 2 months) we need to round up to the nearest 2 month interval. Therefore, the account balance will reach $10,000 after 79 years and 6 months.

Note: After 79 years and 4 months, the account balance will be $9,987.47, and after 79 years and 6 months it will be $10,017.43.

`\hrulefill`

As the account increases by a constant percentage quarterly, we can use the compound interest formula to write a function to model the situation.

`\boxed{\begin{minipage}{8.5 cm}\underline{Compound Interest Formula}\\\\$ A=P\left(1+(r)/(n)\right)^(nt)$\\\\where:\\\\ \phantom{ww}$\bullet$ $A =$ final amount \\ \phantom{ww}$\bullet$ $P =$ principal amount \\ \phantom{ww}$\bullet$ $r =$ interest rate (in decimal form) \\ \phantom{ww}$\bullet$ $n =$ number of times interest is applied per year \\ \phantom{ww}$\bullet$ $t =$ time (in years) \\ \end{minipage}}`

The interest is compounded quarterly, which means every 3 months.

Therefore, the interest is applied 4 times per year.

Given values:

• A = $60,000
• P = $30,000
• r = 4% = 0.04
• n = 4 (quarterly)

Substitute the given values into the formula, and solve for t:

`\begin{aligned}A&=P\left(1+(r)/(n)\right)^(nt)\\\\\implies 60000&=30000\left(1+(0.04)/(4)\right)^(4t)\\\\\implies 60000&=30000\left(1+0.01\right)^(4t)\\\\2&=\left(1.01\right)^(4t)\\\\\textsf{Take natural logs:} \quad \ln \left(2\right)&=\ln\left(1.01\right)^(4t)\\\\\ln \left(2\right)&=4t\ln\left(1.01\right)\\\\t&=(\ln \left(2\right))/(4\ln\left(1.01\right))\\\\t&=17.4151792...\end{aligned}`

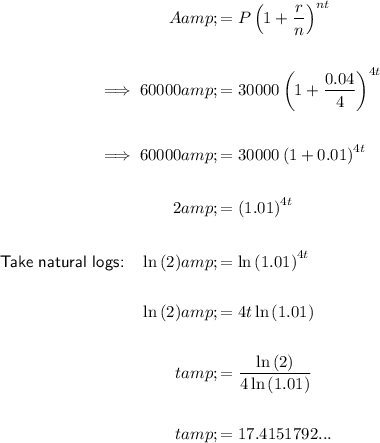
The value of the investment account will double during the 17th year (after 17 years and 4.98 months).

As the interest is applied quarterly (every 3 months) we need to round up to the nearest 3 month interval. Therefore, the investment account will double by 17 years and 6 months.

Note: After 17 years and 3 months, the account balance will be $59,606.83, and after 17 years and 6 months it will be $60,202.90.