Answer:
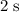 .
.
Step-by-step explanation:
The frequency
 of a wave is the number of cycles completed in unit time.
of a wave is the number of cycles completed in unit time.
In this question, it is given that the frequency of the wave is
 . Thus, on average,
. Thus, on average,
 cycles of this wave are completed in every second.
cycles of this wave are completed in every second.
The period
 of a wave is the time it takes to complete one cycle of the wave.
of a wave is the time it takes to complete one cycle of the wave.
For example, if
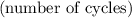 of cycles are completed in a certain
of cycles are completed in a certain
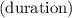 , the time it takes to complete each cycle would be:
, the time it takes to complete each cycle would be:
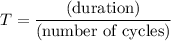 .
.
From the frequency of this wave,
 cycles are completed on average in
cycles are completed on average in
 . Thus,
. Thus,
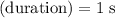 while
while
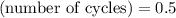 . The time it takes to complete each cycle would be:
. The time it takes to complete each cycle would be:
 .
.
In general, the period of a wave is the reciprocal of frequency:
 .
.
For the wave in this question:
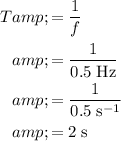 .
.