Question

Help please ! QUESTION 3

If a projectile is fired at 0° from the top of a 100 m building, how long will it take to hit the ground? Assume the initial velocity
of the projectile is 30 m/s.
g= -9.8 m/s²
3.33 s
33 s
20.4 s
4.52 s

Answer 1

The last option, t=4.52 s.

Step-by-step explanation:

The following information will help you tackle any projectile motion problem (assuming there is zero air friction), so please read carefully.

This projectile motion problem is solvable with some basic knowledge of projectile physics and the use of the 4 kinematic equations that are written below.

`\boxed{\left\begin{array}{ccc}\text{\underline{The 4 Kinematic Equations:}}\\\\1. \ \vec v_f=\vec v_0+\vec at\\\\2. \ \Delta \vec x=(1)/(2)(\vec v_f-\vec v_0)t\\\\3. \ \Delta \vec x=\vec v_0t+(1)/(2)\vec at^2\\\\ 4. \ \vec v_f^2=\vec v_0^2+2\vec a \Delta \vec x \end{array}\right}`

Things to note about the above kinematic equations:

In order to use the above equations, two things MUST be true.

i. Acceleration is constant.

ii. You must know at least three pieces of information.

When dealing with 2-D motion, NEVER mix horizontal and vertical components together in the same equation.

Tackling Projectile Motion Problems:

To tackle any projectile motion problem, you will have to split components up into x and y. I personally like to make a table which you will see later as I am solving the question.

Things that are true for all 2-D projectile motion problems:

`\boxed{\left\begin{array}{ccc}\text{\underline{Horizontal Component:}}\\\vec a_x= 0 \ m/s^2\\ \vec v_x=\vec v\cos\theta\end{array}\right} \ \boxed{\left\begin{array}{ccc}\text{\underline{Vertical Component:}}\\\vec a_y= -9.8 \ m/s^2\\\vec v_y=\vec v\sin\theta\end{array}\right}`

The horizontal component of velocity will be the same throughout the projectiles flight while the vertical component of velocity is variable (always changing). The horizontal component of acceleration will always be zero while the vertical component of acceleration will be the acceleration due to gravity. These things are crucial to understand when dealing with projectile motion problems.

~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~

Given:

`\vec v_o=30 \ m/s \ at \ 0 \textdegree\\\\\Delta \vec y= -100 \ m`

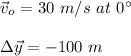
Find:

`t= ?? \ s`

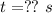
(1) - Splitting up what we know into it horz. and vert. components

`\boxed{\left\begin{array}{ccc}\text{\underline{Horizontal Component:}}\\\vec a_x= 0 \ m/s^2\\ \vec v_(0x)=(30)cos(0 \textdegree)= 30 \ m/s\\\vec v_(fx)= ?? \ m/s\\ \Delta \vec x= ?? \ m\end{array}\right} \ \boxed{\left\begin{array}{ccc}\text{\underline{Vertical Component:}}\\\vec a_y= -9.8 \ m/s^2\\\vec v_(0y)=(30)sin(0 \textdegree)=0 \ m/s\\\vec v_(fy)= ?? \ m/s\\ \Delta \vec y= -100 \ m\end{array}\right}\\\\t=?? \ s`

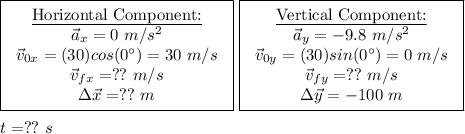
(2) - As you can see from the table above we know three pieces of information for the vertical components. I will use equation 3 to solve for the time, t

`\Delta \vec y=\vec v_(0y)t+(1)/(2)\vec a_yt^2\\\\\Longrightarrow -100=(0)t+(1)/(2)(-9.8)t^2\\\\\Longrightarrow -100=-4.9t^2\\\\\Longrightarrow t^2=20.4082\\\\\Longrightarrow t=√(20.4082) \\\\\therefore \boxed{\boxed{t \approx 4.52 \ s}}`

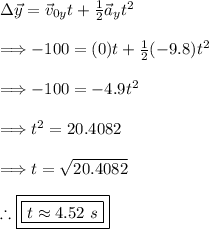
Thus, it takes about 4.52 seconds for the projectile to hit the ground.

Answer 2

Approximately
`4.52\; {\rm s}`, assuming that air resistance on the projectile is negligible.

Step-by-step explanation:

It is given that the initial velocity of the projectile was
`u = 30\; {\rm m\cdot s^(-1)}` with an angle of elevation of
`0^(\circ)`. Decompose this initial velocity into its horizontal and vertical components:

• Horizontal component of initial velocity:
  `u_(x) = u\, \cos(0^(\circ)) = 30\; {\rm m\cdot s^(-1)}`.
• Vertical component of initial velocity:
  `u_(y) = u\, \sin(0^(\circ)) = 0\; {\rm m\cdot s^(-1)}`.

Under the assumptions, the duration of the free fall depends only on the vertical component of initial velocity,
`u_(y) = 0\; {\rm m\cdot s^(-1)}`.

Apply the following SUVAT equation to find this duration:

`\displaystyle x_(y) = (1)/(2)\, a_(y)\, t^(2) + u_(y)\, t`,

Where:

• 
  `x_(y) = (-100)\; {\rm m}` is the vertical displacement of the projectile,
• 
  `a_(y) = g = (-9.8)\; {\rm m\cdot s^(-2)}` is the vertical acceleration of the projectile,
• 
  `t` is the duration of the free fall, and
• 
  `u_(y)` was the initial vertical velocity of the projectile.

Since initial vertical velocity was
`u_(y) = 0\; {\rm m\cdot s^(-1)}`, this equation simplifies to:

`\displaystyle x_(y) = (1)/(2)\, a_(y)\, t^(2)`.

Rearrange this equation to find duration
`t`:

`\begin{aligned}t &= \sqrt{(2\, x_(y))/(a_(y))} \\ &= \sqrt{(2\, (-100))/((-9.8))}\; {\rm s} \\ &\approx 4.52\; {\rm s}\end{aligned}`.