Question

Given sin a = 8/17

Calculate tan a

Answer 1

8/15

Explanation:

Assuming that a is in the 1st quadrant, based on sin a = 8/17 you can build a right triangle as in the picture. Third side you compute with Pythagoras
`x^2 + 8^2 = 17^2 \implies x = 15`.

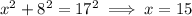At this point the tangent is the ratio of the two segments, or 8/15.

Else, you can calculate cos a based on the fact that
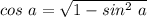`cos\ a = √(1-sin^2\ a)` (under the same assumption as the beginning. Then it's just a matter of plugging numbers.