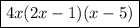Question

Factorise the following ;

1 . x³-x²-6x

2. 3x³- 27x² + 24x

3. 8x³ - 44x² + 20x​

Answer 1

`\textsf{1.} \quad {x(x+2)(x-3)`

`\textsf{2.} \quad 3x(x-1)(x-8)`

`\textsf{3.} \quad 4x(2x-1)(x-5)`

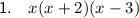
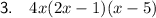
Explanation:

Question 1

To factorize the given cubic expression x³ - x² - 6x, first factor out the common term x:

`x(x^2-x-6)`

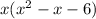
Now factorize the quadratic expression (x² - x - 6) by using the technique of splitting the middle term.

The product of the coefficient of the leading term and the constant is -6. Therefore, find two numbers that multiply to -6 and sum to -1.

The two numbers are -3 and 2, so rewrite the middle term as -3x + 2x:

`\begin{aligned}x^2-x-6&=x^2-3x+2x-6\\&=x(x-3)+2(x-3)\\&=(x+2)(x-3)\end{aligned}`

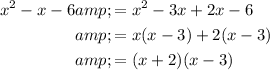
Therefore, the fully factorised expression is:

`\boxed{x(x+2)(x-3)}`

`\hrulefill`

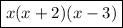
Question 2

To factorize the given cubic expression 3x³- 27x² + 24x, first factor out the common term 3x:

`3x(x^2-9x+8)`

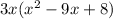
Now factorize the quadratic expression (x² - 9x + 8) by using the technique of splitting the middle term.

The product of the coefficient of the leading term and the constant is 8. Therefore, find two numbers that multiply to 8 and sum to -9.

The two numbers are -8 and -1, so rewrite the middle term as -8x - x:

`\begin{aligned}x^2-9x+8&=x^2-8x-x+8\\&=x(x-8)-1(x-8)\\&=(x-1)(x-8)\end{aligned}`

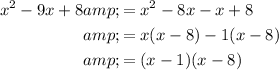
Therefore, the fully factorised expression is:

`\boxed{3x(x-1)(x-8)}`

`\hrulefill`

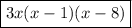
Question 3

To factorize the given cubic expression 8x³ - 44x² + 20x​, first factor out the common term 4x:

`4x(2x^2-11x+5)`

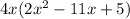
Now factorize the quadratic expression (2x² - 11x + 5) by using the technique of splitting the middle term.

The product of the coefficient of the leading term and the constant is 10. Therefore, find two numbers that multiply to 10 and sum to -11.

The two numbers are -10 and -1, so rewrite the middle term as -10x - x:

`\begin{aligned}2x^2-11x+5&=2x^2-10x-x+5\\&=2x(x-5)-1(x-5)\\&=(2x-1)(x-5)\end{aligned}`

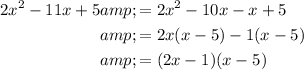
Therefore, the fully factorised expression is:

`\boxed{4x(2x-1)(x-5)}`