Question

Slope intercept equation of (-5,-7)

Answer 1

`\huge\boxed{\sf y=(9)/(5) x + 2}`

Explanation:

Point = (x,y) = (-5, -7)

So,

x = -5, y = -7

General form of slope-intercept equation:

• y = mx + b

Where m is slope and b is y-intercept.

Finding slope:

Along with the already given point, let us take any other point of the line.

Point 1 = (x₁,y₁) = (-5,-7)

Point 2 = (x₂,y₂) = (0,2)

So,

x₁ = -5

y₁ = -7

x₂ = 0

y₂ = 2

`\displaystyle Slope = (y_2-y_1)/(x_2-x_1) \\\\Slope = (2-(-7))/(0-(-5)) \\\\Slope = (2+7)/(0+5) \\\\\boxed{Slope = (9)/(5) }`

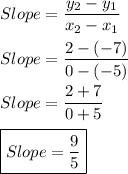
Finding y-intercept:

• y-intercept is actually the point where x = 0.

Here, x = 0 when y = 2.

So,

y-intercept = 2

Put slope and y-intercept in general form of slope-intercept equation.

So,

Answer:

`\displaystyle y=(9)/(5) x + 2\\\\\rule[225]{225}{2}`