Question

What the volue of X makes this equation true? 2(x+7)=4​

Answer 1

• x = -5

Explanation:

`\sf 2\left(x+7\right)=4`

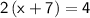
First, let's divide both sides by 2:-

• 
  `\sf \cfrac{2\left(x+7\right)}{2}=\cfrac{4}{2}`

• 
  `\sf x+7=2`

Now, subtract 7 from both sides :-

• 
  `\sf x+7-7=2-7`

• 
  `\sf x=-5`

Therefore, x = -5.

To check if it's true, we plug in the value of x to the equation, If the numbers you get are the same, then the given value is a solution of the equation (makes the equation true). If the numbers don't match, the given value is not a solution of the equation (makes the equation false).

`\sf 2\left(x+7\right)=4`

`\sf x = -5`

• 
  `\sf 2\left((-5)+7\right)=4`

• 
  `\sf 2\left(\left(-5\right)+7\right)=4`

• 
  `\sf 2\cdot \:2=4`

• 
  `4=4`

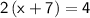
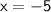
The expressions are the same which makes the equation true.

- - - - - - - - - - - - - - - - - - - - - - - - - - -

Hope this helps!

Answer 2

Answer: -1 1/2.

Explanation:

Step 1: Start by isolating the 'x' factor on one side of the equation.

Step 2: To do this, divide both sides of the equation by 2.

2(x+7)=4

2/2 (x+7) = 4/2

Step 3: Now that the 'x' factor is on one side of the equation, subtract 7 from both sides.

(x+7) - 7 = 4/2 - 7

x = -3/2

Step 4: Follow the order of operations and simplify the value of 'x'.

x = -3/2

x = -1 1/2

Therefore, the value of x that makes this equation true is -1 1/2.