Answer:

Explanation:
Use the point-slope formula (
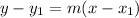 ) to find the slope of the line.
) to find the slope of the line.
Since the line is perpendicular to line L, take the opposite reciprocal of the slope. This means negating the slope and flipping the numerator and denominator.
First, find the slope-intercept form of the line.
5x+2y=18
2y=-5x+18
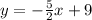
Negate the slope and flip the numerator and denominator. The new slope would be
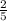 .
.
Now, put the slope and point into the point-slope formula.

Distribute the 2/5.
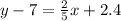
Add 7 to both sides.
