Question

1.

Archaeologists have discovered the legendary Golden Igloo of the Pribiloff Islands, a structure consisting of a

halfspherical shell with an inner diameter of 1.25 m and an out diameter of 1.45 m. What is the value of this antiquity,

given that gold currently sells for $1,263/ounce and has a density of 19.3 g/cm3? (Note that 1 ounce = 31.1034768 g)

Answer 1

The value of the Golden Igloo is $227.4 million.

Step-by-step explanation:

First, we need to find the inner and the outer volume of the half-spherical shell:

`V_(i) = (1)/(2)*(4)/(3)\pi r_(i)^(3)`

`V_(o) = (1)/(2)*(4)/(3)\pi r_(o)^(3)`

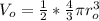
The total volume is given by:

`V_(T) = V_(o) - V_(i)`

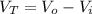
Where:

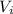`V_(i)`: is the inner volume

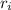`r_(i)`: is the inner radius = 1.25/2 = 0.625 m

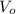`V_(o)`: is the outer volume

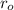`r_(o)`: is the outer radius = 1.45/2 = 0.725 m

Then, the total volume of the Igloo is:

`V_(T) = (2)/(3)\pi r_(o)^(3) - (2)/(3)\pi r_(i)^(3) = (2)/(3)\pi [(0.725 m)^(3) - (0.625 m)^(3)] = 0.29 m^(3)`

Now, by using the density we can find the mass of the Igloo:

`m = 19.3 (g)/(cm^(3))*0.29 m^(3)*((100 cm)^(3))/(1 m^(3)) = 5.60 \cdot 10^(6) g`

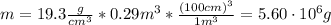
Finally, the value (V) of the antiquity is:

`V = (\$ 1263)/(oz)*5.60 \cdot 10^(6) g*(1 oz)/(31.1034768 g) = \$ 227.4 \cdot 10^(6)`

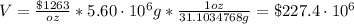
Therefore, the value of the Golden Igloo is $227.4 million.

I hope it helps you!