Question

2. The base of a solid is a region bounded by the curve x^2/8^2+y^2/4^2=1(an ellipse with the major and minor axes of lengths 16 and 8 respectively). Find the volume of the solid if every cross section by a plane perpendicular to the major axis (x-axis) has the shape of an isosceles triangle with height equal to 1/4 the length of the base

Answer 1

85 1/3 cubic units

Explanation:

You want the volume of a solid whose base is the ellipse in the x-y plane with axes 16 and 8 units and whose cross sections perpendicular to the major axis are isosceles triangles with height equal to 1/4 of the cross section dimension parallel to the minor axis.

Area

The isosceles triangle cross section has base 2y and height (2y)/4, so area of ...

A = 1/2bh

A = (1/2)(2y)(2y/4) = y²/2

Volume

A differential of volume is the product of the area of a slice and its thickness:

dV = A·dx = (1/2)y²·dx

The total volume is the integral of this differential of volume over the interval x ∈ [-8, 8]. That integral will be twice the integral on the interval [0, 8}, so we have ...

`\displaystyle V=2\int_0^8{(y^2)/(2)}\,dx=16\int_0^8{\left(1-(x^2)/(64)\right)}\,dx=16\left((8-0)-(8^3-0^3)/(3\cdot64)\right)\\\\\boxed{V=85(1)/(3)\text{ cubic units}}`

__

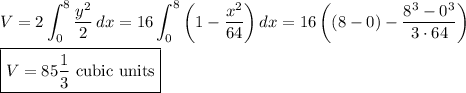
Alternate computation

The volume of half an ellipsoid with semi-axes 2, 4, and 8 is ...

V = (1/2)(4/3)π(abc)

V = (1/2)(4/3)π(2)(4)(8) = 128π/3

The area of half an ellipse with semi-axes 2 and 4 is ...

A = (1/2)π(ab)

A = (1/2)π(2)(4) = 4π

The area of a triangle with the same semi-axes is ...

A = 1/2bh = (1/2)(2)(2·4) = 8

Then the ratio of triangle area to ellipse area at each cross section is ...

triangle / ellipse = 8/(4π) = 2/π

The volume of the solid of interest is estimated to be this factor multiplied by the volume of the half-ellipsoid:

(2/π)(128π/3) = 256/3 = 85 1/3 . . . . same as above