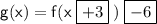Question

NO LINKS!!!! URGENT HELP PLEASE!!!

Please help me with these problems

Answer 1

`\sf g(x) = f(x\; \boxed{+ 3}\;) \;\boxed{- 6}`

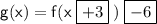
Explanation:

A translation is a transformation that moves every point of a figure the same distance and in the same direction. This means that the size, shape, and orientation of the figure are preserved, but its position is changed.

From inspection of the given diagram, we can see that the size, shape and orientation of the graph of function g is the same as that of the graph of function f, but its position has changed. Therefore, the transformation is a translation.

We can use the vertex of both graphs to determine the translation.

• The vertex of function f is the origin (0, 0).
• The vertex of function g is (-3, -6).

Therefore, the graph of function f has been moved 3 units left and 6 units down to create the graph of function g.

When we move "a" units left, we add the value of "a" to the x-value of the function.

When we move "a" units down, we subtract the value of "a" from the function.

Therefore:

`\sf g(x) = f(x\; \boxed{+ 3}\;) \;\boxed{- 6}`