Question

50 PTS !! PLEASE HELPPP <33

Answer 1

Sally is the answer

Step-by-step explanation:

Apply

x^m/n = n √ x^m

to rewrite the exponentiation as a radical.

Answer 2

Sally √(x³)

Step-by-step explanation:

You want to write x^(3/2) using a radical symbol.

Root

It helps to remember a couple of the rules of roots and exponents.

If 'a' is a square root of x, this means ...

a·a = x

Remembering that (a^b)^c = a^(bc), we can write this as ...

`a\cdot a=a^2\\\\a^2=x\\\\(a^2)^(1)/(2)=x^(1)/(2)\qquad\text{both to $(1)/(2)$ power}\\\\a^{2\cdot(1)/(2)}=x^(1)/(2)\\\\a^1=x^(1)/(2)\\\\a=x^(1)/(2)\\\\√(x)=x^(1)/(2)\qquad\textsf{$a$ is a square root of $x$}`

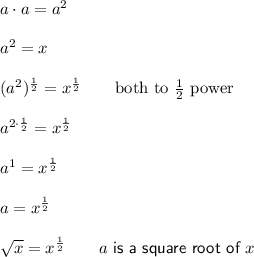
Perhaps you can see that this works with any root index. That is, for example, ...

`\sqrt[3]{x}=x^(1)/(3)`

Numerator

As we saw above, the square of the square root is the original number. The power (2) multiplied the exponent representing the root (1/2) to give an exponent of 1.

If the power is 3 instead of 2, the same thing happens.

`x^(3)/(2)=(x^3)^(1)/(2)=\boxed{√(x^3)\qquad\textsf{matches Sally's rewrite}}`